Next: Other classes of categories Up: An historical survey of Previous: An historical survey of
Digression: Tarski's High School Algebra Problem
It would be unfair here not to give at least a brief description of the works
in number theory that made possible this
first elegant characterization of the isomorphisms that hold in all
ccc's. We will give here a general overview of the works in this field known to
the author, as well as a list of references for the interested reader.
In [DT69], Tarski first asked if the
following usual equalities (called
theory  ) that are taught in high school are complete
for the natural numbers (i.e., if they are enough to prove all the true
numerical equalities):
) that are taught in high school are complete
for the natural numbers (i.e., if they are enough to prove all the true
numerical equalities):

He conjectured that they were enough,![]() but was
not able to prove his conjecture. His student Martin could show that
but was
not able to prove his conjecture. His student Martin could show that
 is complete for
is complete for 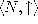 ,
and also that
,
and also that
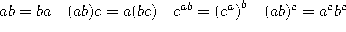 are
complete for
are
complete for 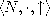 , but he exhibited a simple valid
equation involving sums that is not provable from these axioms:
, but he exhibited a simple valid
equation involving sums that is not provable from these axioms:![]()
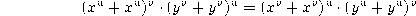
The question, though, was not settled by this counterexample, because it is a counterexample only if we do not allow a constant for 1, that Tarski clearly considered necessary, even if he did not explicitly mention it in his conjecture. In the presence of a constant 1, the following new equations come into play, and allow us to easily prove Martin's equality.
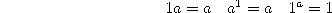
This problem attracted the interest of many other mathematicians, like Leon
Henkin, who focused on the equalities valid in 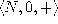 , and
showed the completeness of the usual known axioms (commutativity, associativity
of the sum and the zero axiom), and gives a very nice presentation of the topic
in [Hen77].
, and
showed the completeness of the usual known axioms (commutativity, associativity
of the sum and the zero axiom), and gives a very nice presentation of the topic
in [Hen77].
Wilkie was the first to show with a proof theoretic approach in [Wil81] that even with 1 and the new axioms that come with 1, there are true identities that are not provable, like

where A=(x+1), 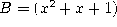 ,
, 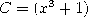 ,
,  .
.
Gurevic later gave a simpler model theoretic proof of this
fact [Gur85] and showed finally that for the valid equalities of
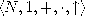 there is no finite axiomatization , by
means of an indexed family of equalities that generalize Wilkie's
identity [Gur90].
Nonetheless, equality in all these structures, even if not finitely
axiomatizable, is decidable [Mac81, Gur85].
there is no finite axiomatization , by
means of an indexed family of equalities that generalize Wilkie's
identity [Gur90].
Nonetheless, equality in all these structures, even if not finitely
axiomatizable, is decidable [Mac81, Gur85].![]()
As often happens in number theory, these last results use far more complex tools
than simple arithmetic reasoning, as in the case of [HR84], where
Nevanlinna theory is used to identify a subclass of numerical expressions for
which the usual axioms for +,  ,
, 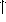 and 1 are complete.
and 1 are complete.
This remarkable analogy between isomorphisms and equalities in number theory works quite well for the first-order case, even if it seems quite hard to find an extension to higher-order languages.
Next: Other classes of categories Up: An historical survey of Previous: An historical survey of Roberto Di Cosmo
Mon Aug 30 11:21:44 MET DST 1999
